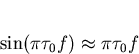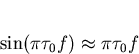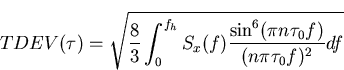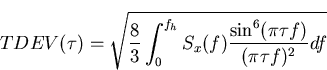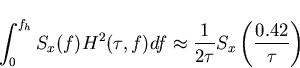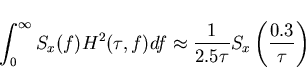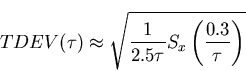Next: TDEV Response to Different
Up: Selection of TDEV as
Previous: TDEV in terms of
A useful approximation to make equation (4.6) more analytically tractable has been obtained by Dan Wolaver of Tektronix Ltd.[19].
For  small to avoid anti-aliasing,
small to avoid anti-aliasing,
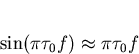
over the range of integration  .Thus equation (4.6) becomes
.Thus equation (4.6) becomes
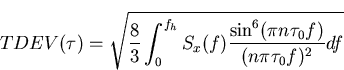
which since  , simplifies to
, simplifies to
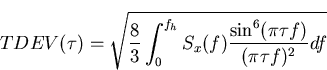
Thus TDEV may be approximated by
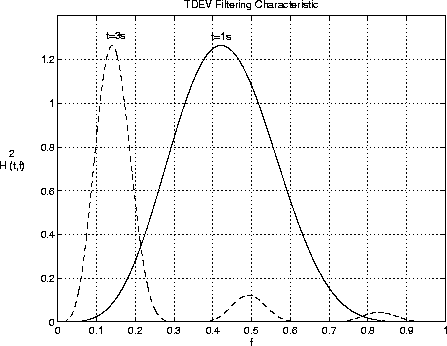
From the above equation, TDEV is the rms of phase filtered by  . This function is plotted in figure 4.3 for
. This function is plotted in figure 4.3 for 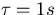 and
and 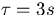 . It can be seen
. It can be seen  is nearly zero except near
is nearly zero except near 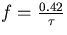 and that the filter can be loosely approximated to a bandpass filter centred on
and that the filter can be loosely approximated to a bandpass filter centred on  .
.
Figure 4.3:
Frequency Response of TDEV Filter for 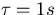 and
and 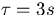
 |
Since the area under the curve is 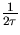 , one might use the approximation
, one might use the approximation
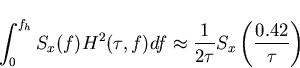
In actual fact, the bandpass is very wide and because dominant noise sources such as flicker phase (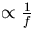 ) etc. have strongest spectral components at low frequencies, the approximation
) etc. have strongest spectral components at low frequencies, the approximation
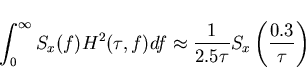
is more appropriate and has been found [20] to be within 20% of the exact value for observation intervals up to 106 seconds.
Thus TDEV may be approximated by
| 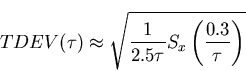 |
(31) |




Next: TDEV Response to Different
Up: Selection of TDEV as
Previous: TDEV in terms of
Mark J Ivens
11/13/1997